Parents play a key role in getting children interested in Science. Science is a living subject that surrounds us everyday. There’s Science in the kitchen, the bathroom, the living room, in fact, everywhere. The following are some questions that children typically ask, or questions that parents can ask their children, so as to set their minds thinking:
(1) Why do metallic objects feel colder than wooden ones?
(2) Why does ice feel cold?
(3) Why is the air-con unit placed near the ceiling?
(4) Why do we feel cold just after taking a bath?
(5) Why does oil float on water?
(6) Why does ice float on water?
(7) Why do metal ships float on water?
(8) Why does the swimming pool appear shallower than it really is?
(9) How does a pair of spectacles work?
(10) How does a fan work?
(11) Why do we need to breathe air?
(12) Why do oranges and lemons taste sour?
(13) Why do salt and sugar melt faster in warmer water?
(14) What causes the tides in the sea?
(15) Why are we able to see the moon?
(16) Why do moving toys need batteries? What goes on in a dry cell?
(17) Why does food last longer when frozen?
(18) Can a see-saw be balanced when two people are sitting on it? How?
(19) Why does the swing stop after some time?
(20) ……………..
There are many more such questions that parents and children can think of. These questions have both vague, unscientific answers as well as the scientific ones. It is understandable that most parents have forgotten the correct answers to these questions, but this should not deter parents from learning with their children.
It would be a good idea for parents to buy or borrow a basic science encyclopedia to read with their children. Searching for information together on the internet is also a good joint activity between parents and children.
The true nature of Science is learning by thinking and exploring, observing and experimenting, making many errors along the way. It is this spirit of thinking and persistence that gives us many of the useful things that we have today, like electricity and aeroplanes. Teenagers can certainly afford to spend some time thinking about how computers or hand phones work, instead of just using them.
Ilyasa, M.Ed, PGDE, B.Sc, ex-sch teacher.
Teaching Math to kids everyday ….
I believe the human brain has a math processor that needs to be activated and nurtured regularly. Again, parents play an important role in making a child comfortable with numbers and calculations. The following are some concepts in daily life that deal with math:
(1) Time: Parents can ask children to add hours and mins to the current time to arrive at a specific time for some activity. Alternatively, give the child a time problem that he needs to solve by working backwards. For example, “if we want to reach the shopping mall at 5:15pm and we take 40 mins to get there, at what time should we leave the house?”
(2) Money: Counting money, calculating change (remainder), determining the price after discount, budgeting and calculating profit or loss are some of the mathematical activities that we may encounter daily or weekly.
(3) Shapes and figures: In toys, art and household furniture there are shapes and figures for children to think about. How about the area and perimeter of such shapes? How about the volumes of some 3-D figures? The concepts of proportion, similarity and congruency can also be discussed when observing shapes. For example, two dining table chairs are congruent. A baby elephant and a mother elephant are similar but not congruent.
(4) Lines and gradient: Look around us and we can see many parallel lines, intersecting lines and perpendicular lines. How about the slope (gradient) of a road outside the house? The steepness of a staircase?
(5) Distance and speed: What is speed? How is it determined? Observe walking speeds, cycling speeds and the speeds of vehicles. While taking a taxi, estimate its average speed and take note of the travelling time. Estimate how far the taxi travelled.
(6) Statistics: In the newspapers we can find pie charts and bar charts, and the terms “average” and “mean”. What do the charts show and what do these terms mean? Even young kids can interpret pie charts. Parents can relate pie charts to cutting a birthday cake.
There are many more examples of mathematics in daily life. When children understand cost price, selling price, revenue and profit, they are on their way to understanding business and the economy. Entrepreneurship can then develop from there.
Ilyasa, M.Ed, PGDE, B.Sc.
Metacognition enhances learning
Various studies have revealed that metacognition helps to enrich students’ learning in different domains. For example, it has the potential to increase students’ capacities for independent learning (Ganz & Ganz, 1990).
Research also shows that knowledge of metacognition, such as being familiar with one’s strengths and weaknesses and searching for ways to overcome the latter, contributes to more effective learning (Bransford, Brown, & Cocking, 1999). Research also suggests that metacognition improves one’s chances of success when it comes to completing activities that rely heavily on thinking processes (Garner & Alexander, 1989; Pressley & Ghatala, 1990).
Many studies in metacognition have concluded that those who have advanced metacognitive abilities are more adaptable and steadfast in problem solving (e.g., see Artzt & Armour-Thomas, 1992; Swanson, 1990). Studies have also shown that one’s ability to plan and monitor a problem-solving process requires several metacognitive skills such as regulation and evaluation of thought processes (Mayer, 1999), and the use of metacognitive skills has the potential to identify the more able students from the less able ones (Pellegrino, Chudowsky & Glaser, 2001).
In addition, research has shown that one’s individual and group learning skills can be improved through the acquisition of metacognitive competencies (White & Frederiksen, 2005). Recent studies have also revealed that students who often fail to choose appropriate strategies, monitor or regulate their work, or articulate their thought processes are more likely to perform poorly in mathematics (e.g., see Lucangeli & Cabrele, 2006; Carlson & Bloom, 2005).
Note: The above paragraphs are adapted from my minor research paper, Examining Supports for Metacognition in Singaporean Lower Secondary Mathematics Textbooks, NIE, 2011. All rights reserved.
Related links:
(1) Metacognition – The secret to learning and problem-solving;
(2) Metacognition and problem-solving;
(3) Is Metacognition part of the Singapore Math curriculum?
_______________________________________
TUITION CLASSES:
_______________________________________________________________
EDUCATIONAL SERVICES:
______________________________________________________________
By EX-MOE TEACHERS & EXPERIENCED TUTORS
@ BLK 644, BUKIT BATOK CENTRAL, #01-68. S(650644).
CALL 65694897 OR SMS 98530744 OR 97860411.
Metacognition and mathematical problem solving
Metacognition is a crucial element in problem-solving, which is itself a key component in mathematics learning. To monitor and regulate one’s cognitive processes in problem-solving, Polya (1945) describes a four-step method: first, one has to comprehend the problem by sub-dividing it into more manageable parts and recognize any given data, conditions and variables to be found; second, one devises or selects a strategy to find the connections between the known data and the unknowns to be found; third, one executes the plan, scanning, regulating and examining each step; and, finally, after obtaining the solution, one evaluates the results which may involve re-visiting the previously taken steps.
Building on Polya’s work, Schoenfeld (1987) describes effective mathematical problem-solving as being contingent on how one uses four types of knowledge/skills: (1) resource knowledge, which is knowledge about one’s abilities and cognitive processes including knowledge of how to perform tasks or procedures; (2) heuristics, which are specific problem-solving methods or strategies; (3) regulatory processes, which includes the organisation and selection of resources and strategies; and (4) beliefs, which includes perceptions of and assumptions about mathematics (Gama, 2004).
Note: The above paragraphs are adapted from my minor research paper, Examining Supports for Metacognition in Singaporean Lower Secondary Mathematics Textbooks, NIE, 2011. All rights reserved.
Related links:
(1) Metacognition – The secret to learning and problem-solving;
(2) Metacognition enhances learning;
(3) Is Metacognition part of the Singapore Math curriculum?
_______________________________________
TUITION CLASSES:
_______________________________________________________________
EDUCATIONAL SERVICES:
______________________________________________________________
By EX-MOE TEACHERS & EXPERIENCED TUTORS
@ BLK 644, BUKIT BATOK CENTRAL, #01-68. S(650644).
CALL 65694897 OR SMS 98530744 OR 97860411.
About Concept Learning @ Jurong West
For our latest timetable, click here => 
=============================================================
ORIGINAL POST (OUTDATED):

PSLE MATH & SCIENCE – NEW CLASSES!
For our latest timetable, click here => 
=============================================================
ORIGINAL POST (OUTDATED):
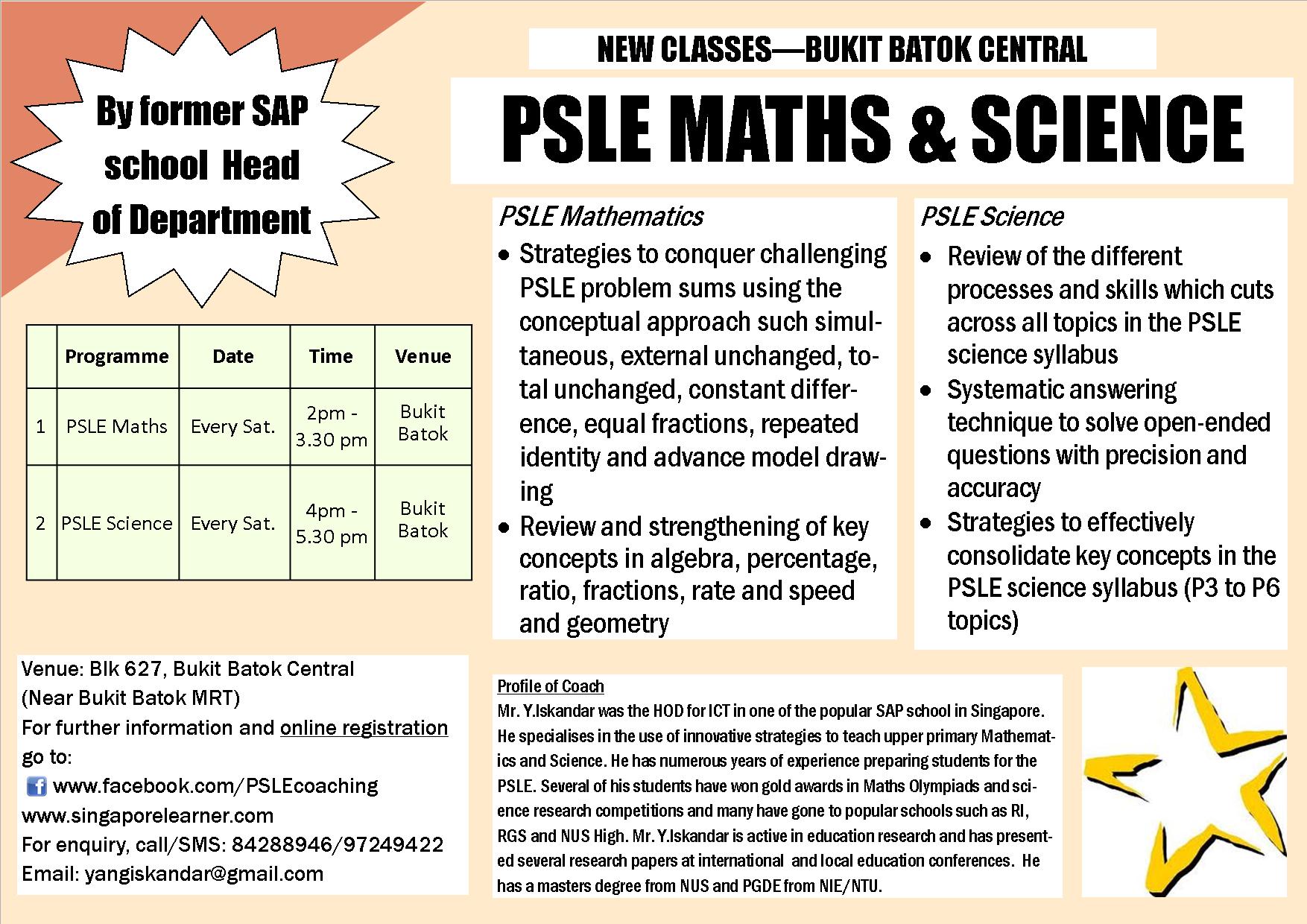
Click here to register for the above programme.
For further information and enquiries please go to facebook.com/pslecoaching






